1.2 — Technology and Cost
ECON 326 • Industrial Organization • Spring 2023
Ryan Safner
Associate Professor of Economics
safner@hood.edu
ryansafner/ioS23
ioS23.classes.ryansafner.com
This Black Box We Call "Firms"
Firm is a mere production process:
- a bundle of technology, physical assets, and individuals
Synonymous with production function
Fully replicable
We'll explore (and explode) this much later
What Do Firms Do? I
We'll assume “the firm” is the agent to model:
So what do firms do?
How would we set up an optimization model:
Choose: < some alternative >
In order to maximize: < some objective >
Subject to: < some constraints >
What Do Firms Do? II
- Firms convert some goods to other goods:

What Do Firms Do? II
Firms convert some goods to other goods:
Inputs: x1,x2,⋯,xn
- Examples: worker efforts, warehouse space, electricity, loans, oil, cardboard, fertilizer, computers, software programs, etc

What Do Firms Do? II
Firms convert some goods to other goods:
Inputs: x1,x2,⋯,xn
- Examples: worker efforts, warehouse space, electricity, loans, oil, cardboard, fertilizer, computers, software programs, etc
Output: q
- Examples: gas, cars, legal services, mobile apps, vegetables, consulting advice, financial reports, etc

What Do Firms Do? III
- Technology or a production function: rate at which firm can convert specified inputs (x1,x2,⋯,xn) into output (q) q=f(x1,x2,⋯,xn)

Production Function as Recipe
The production function
The production algorithm
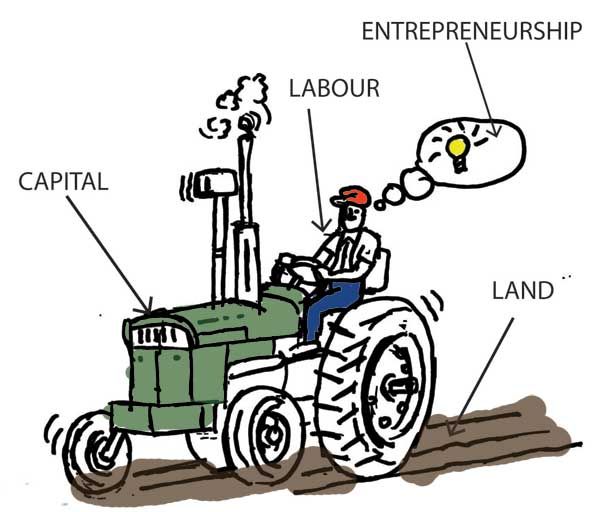
Factors of Production I
q=Af(t,l,k)
- Economists typically classify inputs, called the “factors of production” (FOP):
| Factor | Owned By | Earns |
|---|---|---|
| Land (t) | Landowners | Rent |
| Labor (l) | Laborers | Wages |
| Capital (k) | Capitalists | Interest |
- A: "total factor productivity" (ideas/knowledge/institutions)
Factors of Production II
q=f(l,k)
- We will assume just two inputs: labor l and capital k
| Factor | Owned By | Earns |
|---|---|---|
| Labor (l) | Laborers | Wages |
| Capital (k) | Capitalists | Interest |

What Does a Firm Maximize?
We assume firms maximize profit (π)
Not true for all firms
- Examples: non-profits, charities, civic associations, government agencies, criminal organizations, etc
Even profit-seeking firms may also want to maximize additional things
- Examples: goodwill, sustainability, social responsibility, etc
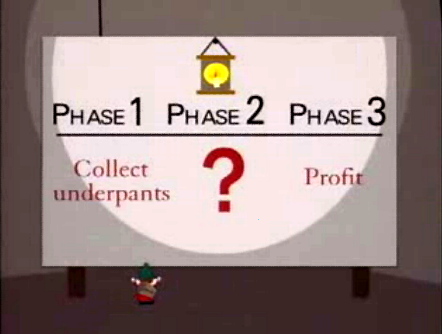
Profits Have a Bad Rap These Days

What is Profit?
- In economics, profit is simply benefits minus (opportunity) costs

What is Profit?
In economics, profit is simply benefits minus (opportunity) costs
Suppose firm sells output q at price p

What is Profit?
In economics, profit is simply benefits minus (opportunity) costs
Suppose firm sells output q at price p
It can buy each input xi at an associated price pi, i.e.
- labor l at wage rate w
- capital k at rental rate r

What is Profit?
In economics, profit is simply benefits minus (opportunity) costs
Suppose firm sells output q at price p
It can buy each input xi at an associated price pi, i.e.
- labor l at wage rate w
- capital k at rental rate r
The profit of selling q units and using inputs l,k is:

Who Gets the Profits? I
π=pqrevenues−(wl+rk)costs

Reminder from Macroeconomics: “The Circular Flow”
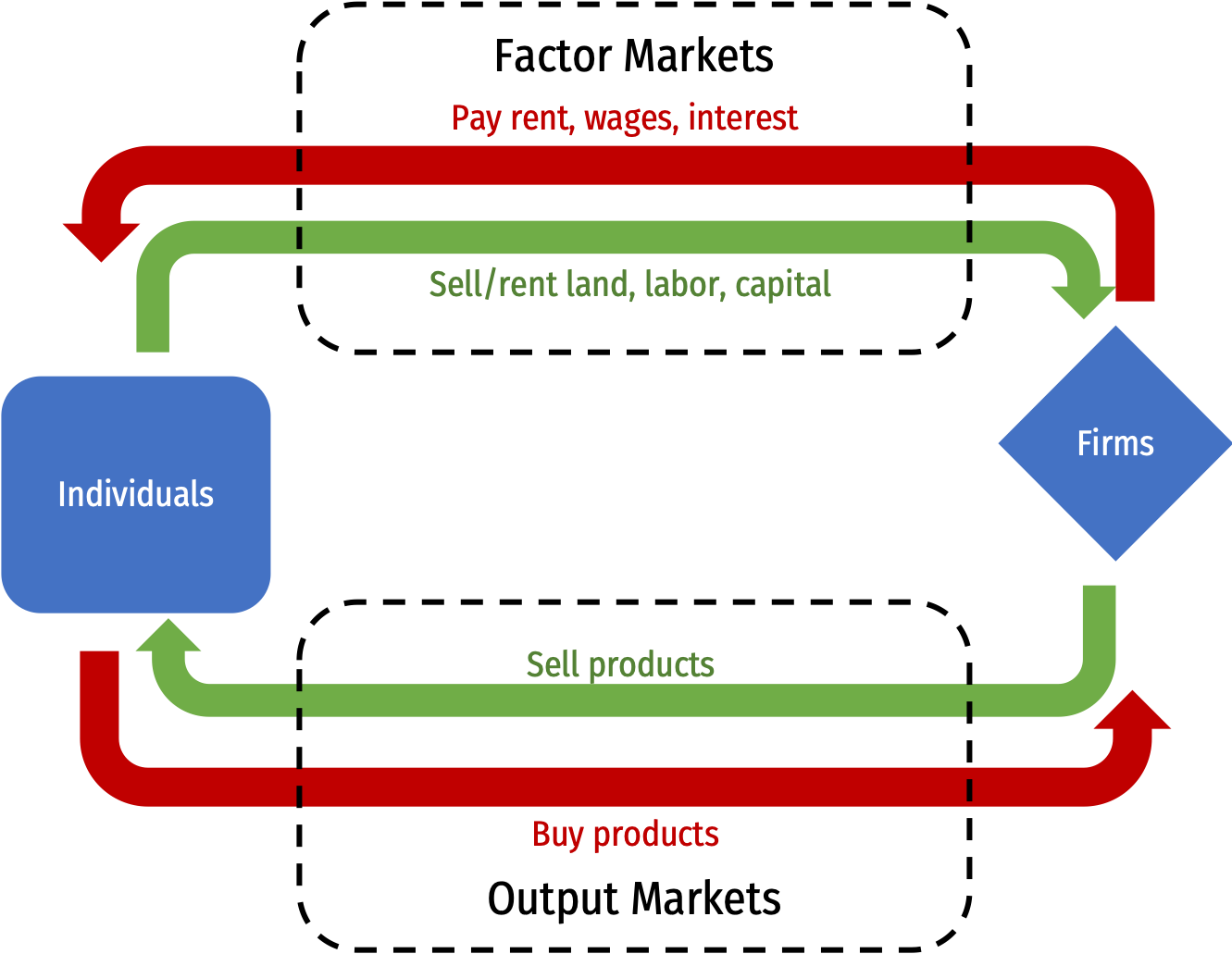
Who Gets the Profits? I
π=pqrevenues−(wl+rk)costs
- The firm's costs are all of the factor-owner's incomes!
- Landowners, laborers, creditors are all paid rent, wages, and interest, respectively

Who Gets the Profits? I
π=pqrevenues−(wl+rk)costs
Profits are the residual value leftover after paying all factors
Profits are income for the residual claimant(s) of the production process (i.e. owner(s) of a firm):
- Entrepreneurs
- Shareholders

Who Gets the Profits? II
π=pqrevenues−(wl+rk)costs
Residual claimants have incentives to maximize firm's profits, as this maximizes their own income
Entrepreneurs and shareholders are the only participants in production that are not guaranteed an income!
- Starting and owning a firm is inherently risky!

Profits and Entrepreneurship: A Preview
In markets, production must face the profit test:
- Is consumer's willingness to pay > opportunity cost of inputs?
Profits are an indication that value is being created for society
Losses are an indication that value is being destroyed for society
Survival in markets requires firms continually create value & earn profits

The Firm's Optimization Problem I
- So what do firms do?
Choose: < some alternative >
In order to maximize: < profits >
Subject to: < technology >
- We've so far assumed they maximize profits and they are limited by their technology

The Firm's Optimization Problem II
What do firms choose? (Not an easy answer)
Prices?
- Depends on the market the firm is operating in!
- Study of industrial organization
Essential question: how competitive is a market? This will influence what firms (can) do

Short-Run Production Concepts
Marginal Products
The marginal product of an input is the additional output produced by one more unit of that input (holding all other inputs constant)
Like marginal utility
Similar to marginal utilities, I will give you the marginal product equations
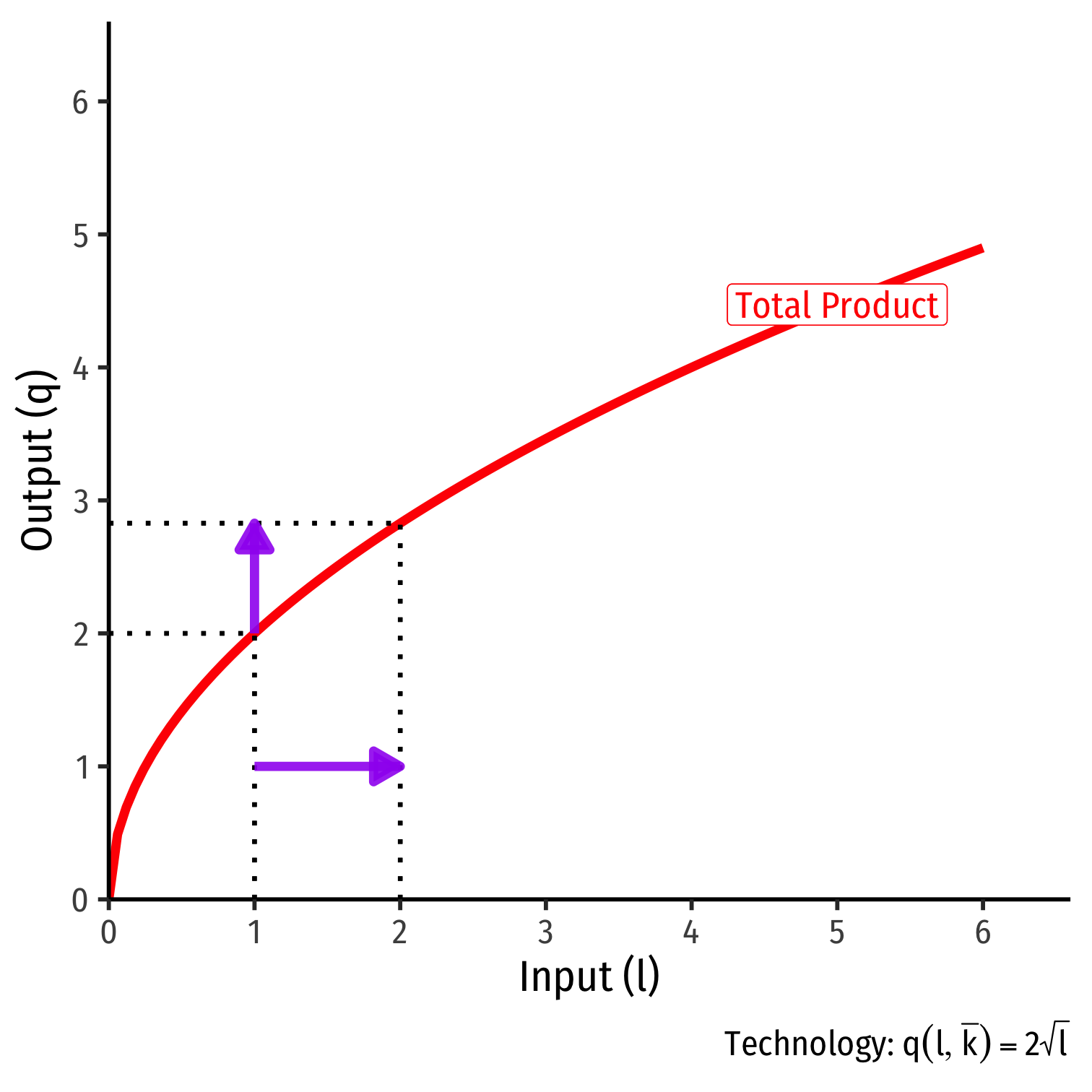
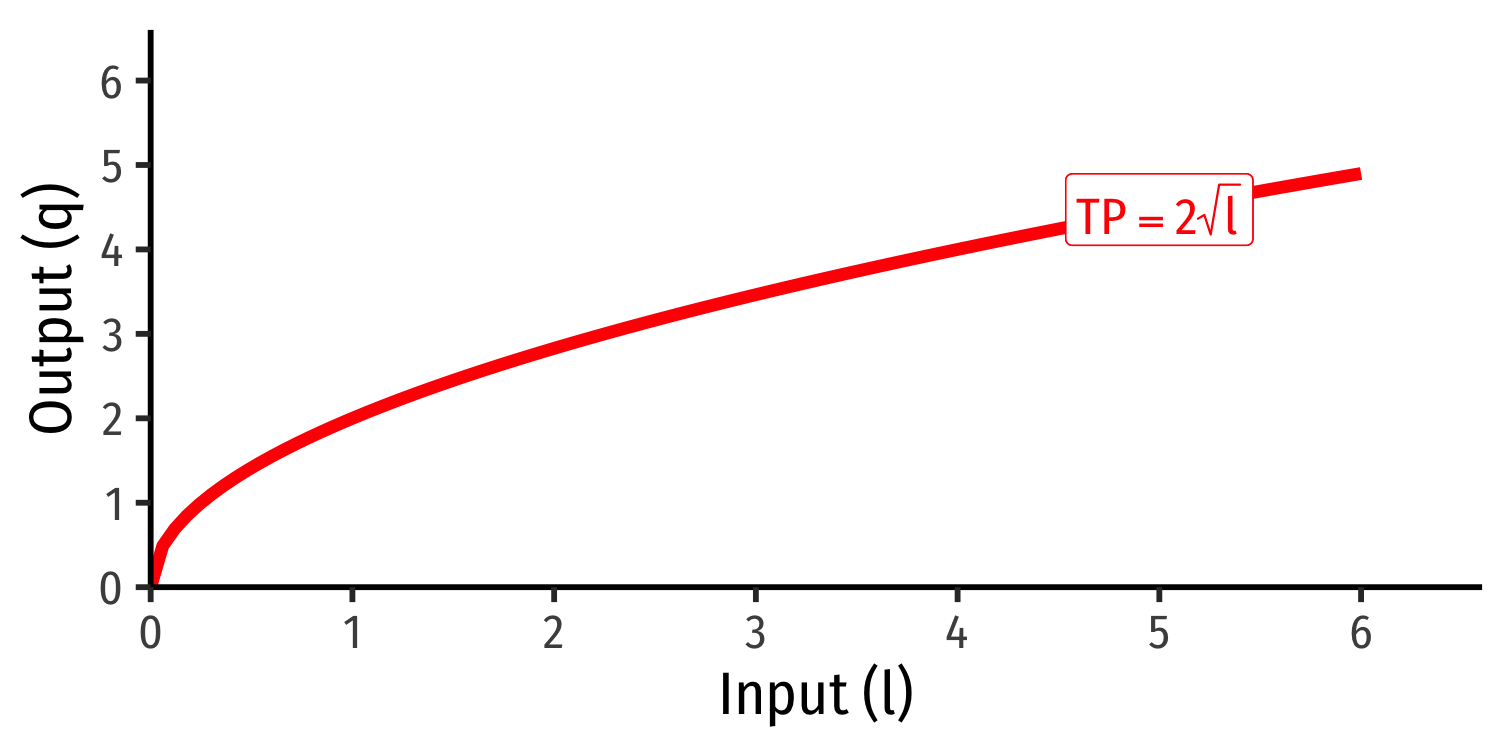
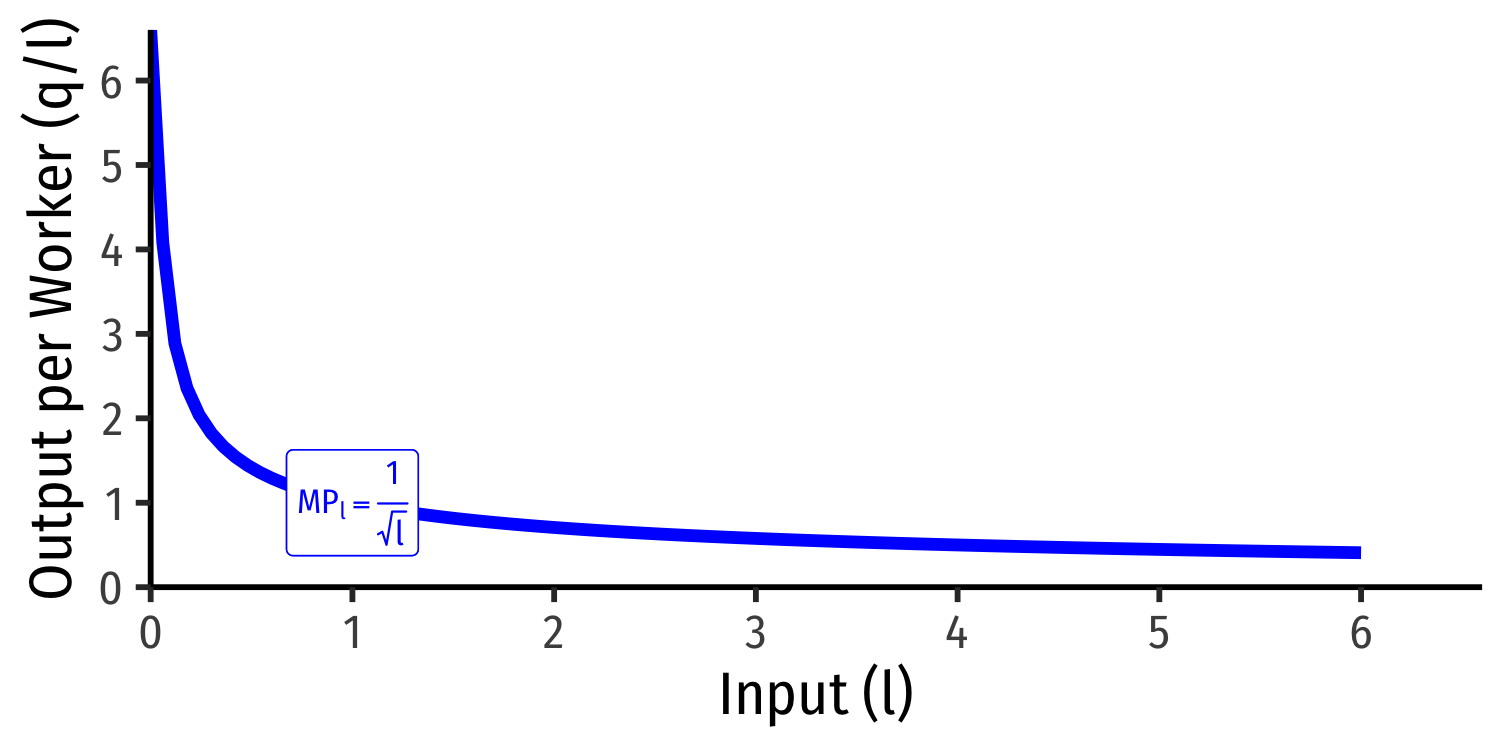
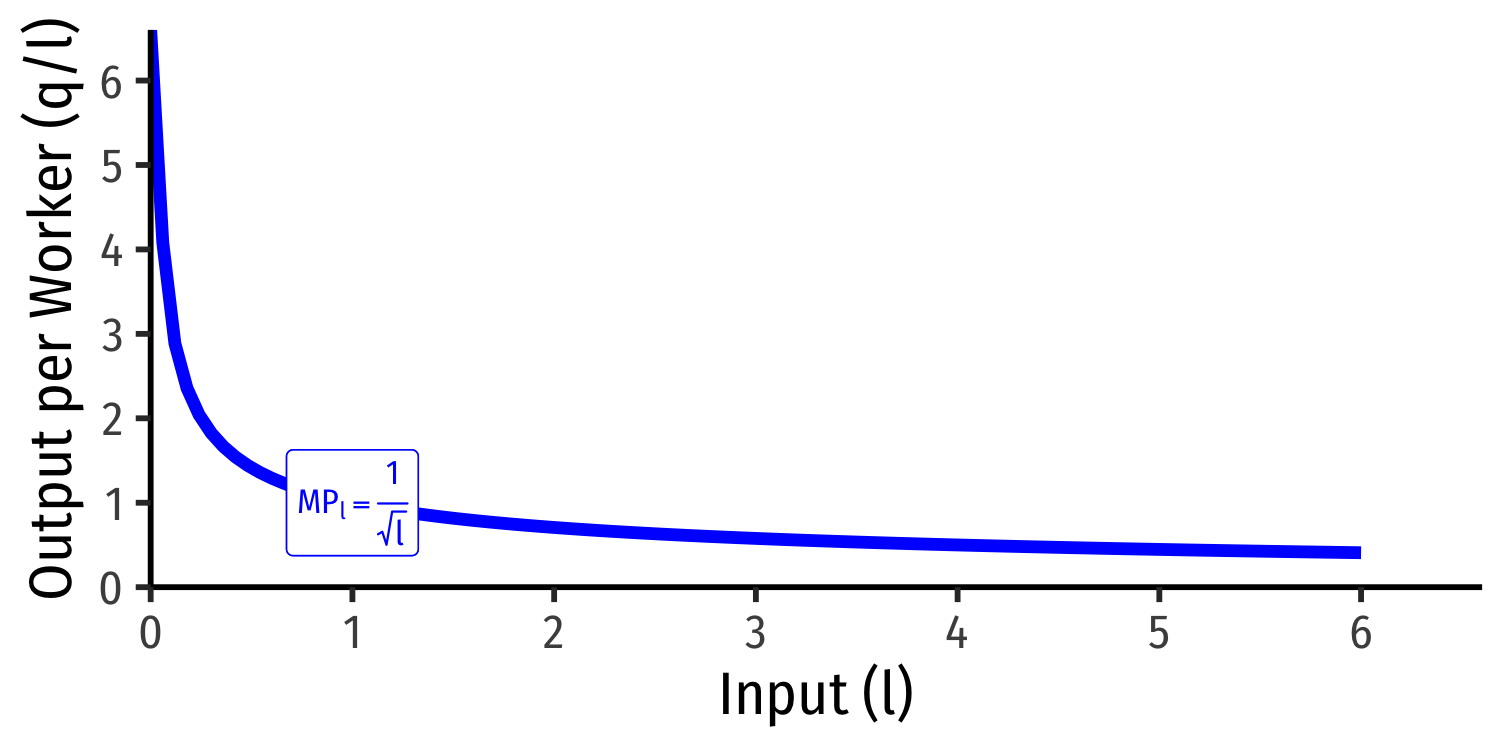
Marginal Product of Labor
Marginal product of labor (MPl): additional output produced by adding one more unit of labor (holding k constant) MPl=ΔqΔl
MPl is slope of TP at each value of l!
- Note: via calculus: ∂q∂l
Marginal Product of Capital
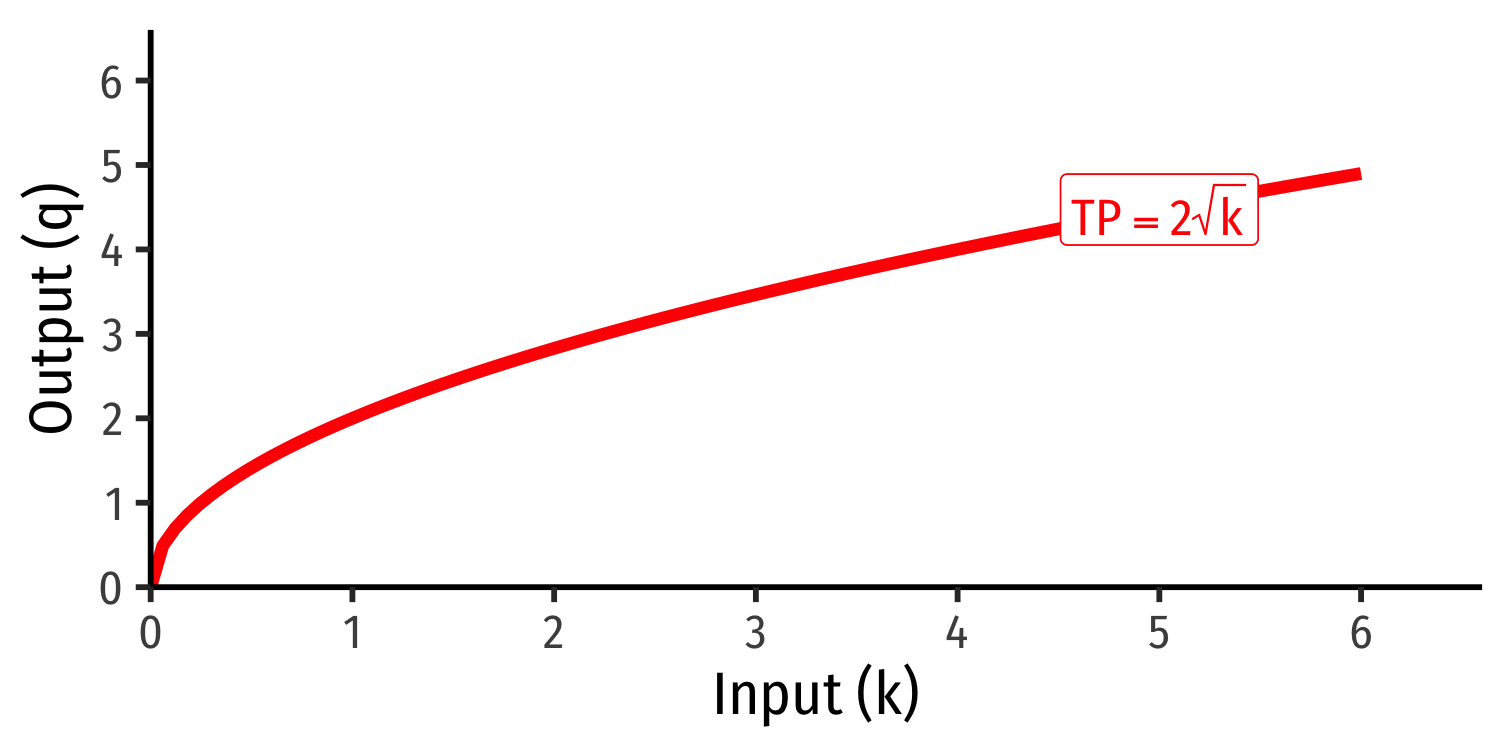
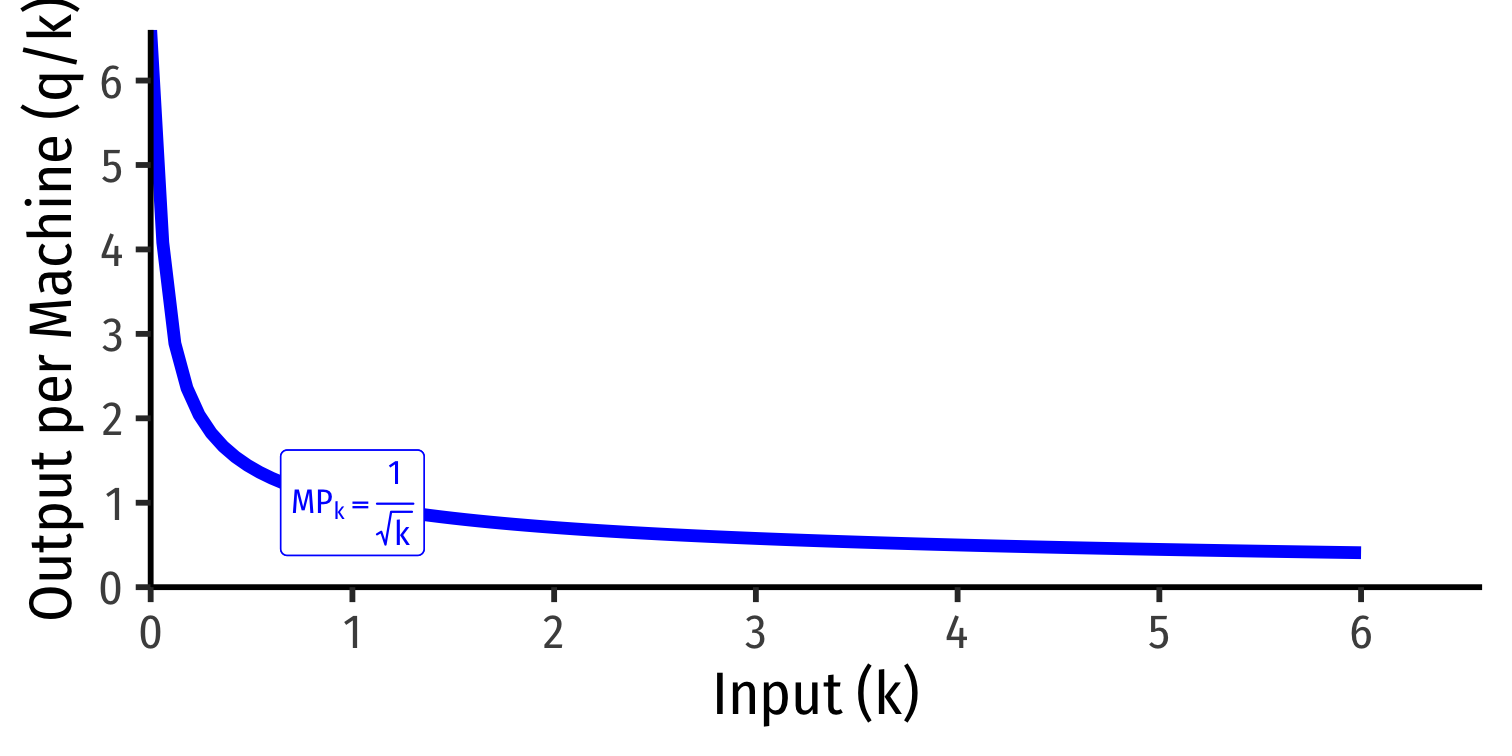
Marginal product of capital (MPk): additional output produced by adding one more unit of capital (holding l constant) MPk=ΔqΔk
MPk is slope of TP at each value of k!
- Note: via calculus: ∂q∂k
Note we don't consider capital in the short run!
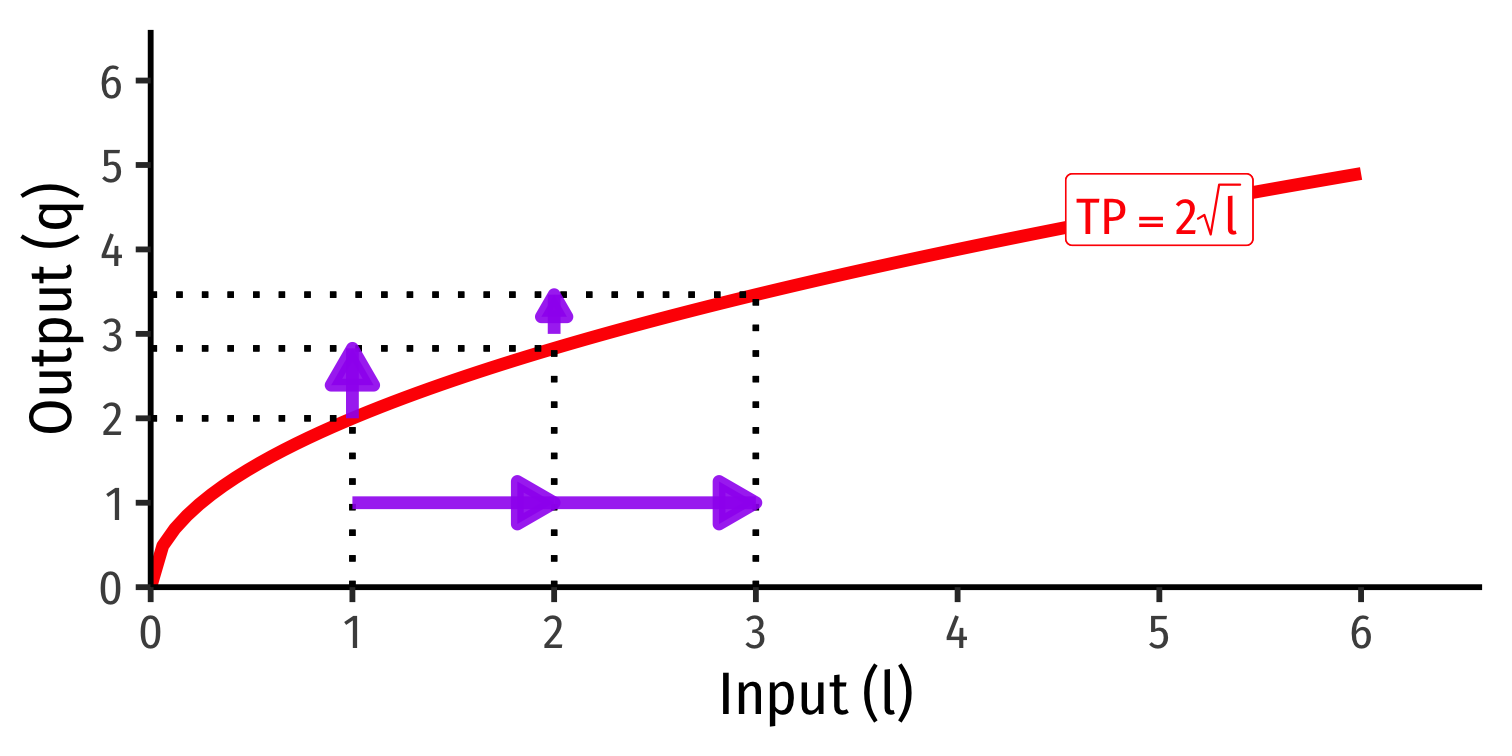
Diminishing Returns
Law of Diminishing Returns: adding more of one factor of production holding all others constant will result in successively lower increases in output
In order to increase output, firm will need to increase all factors!
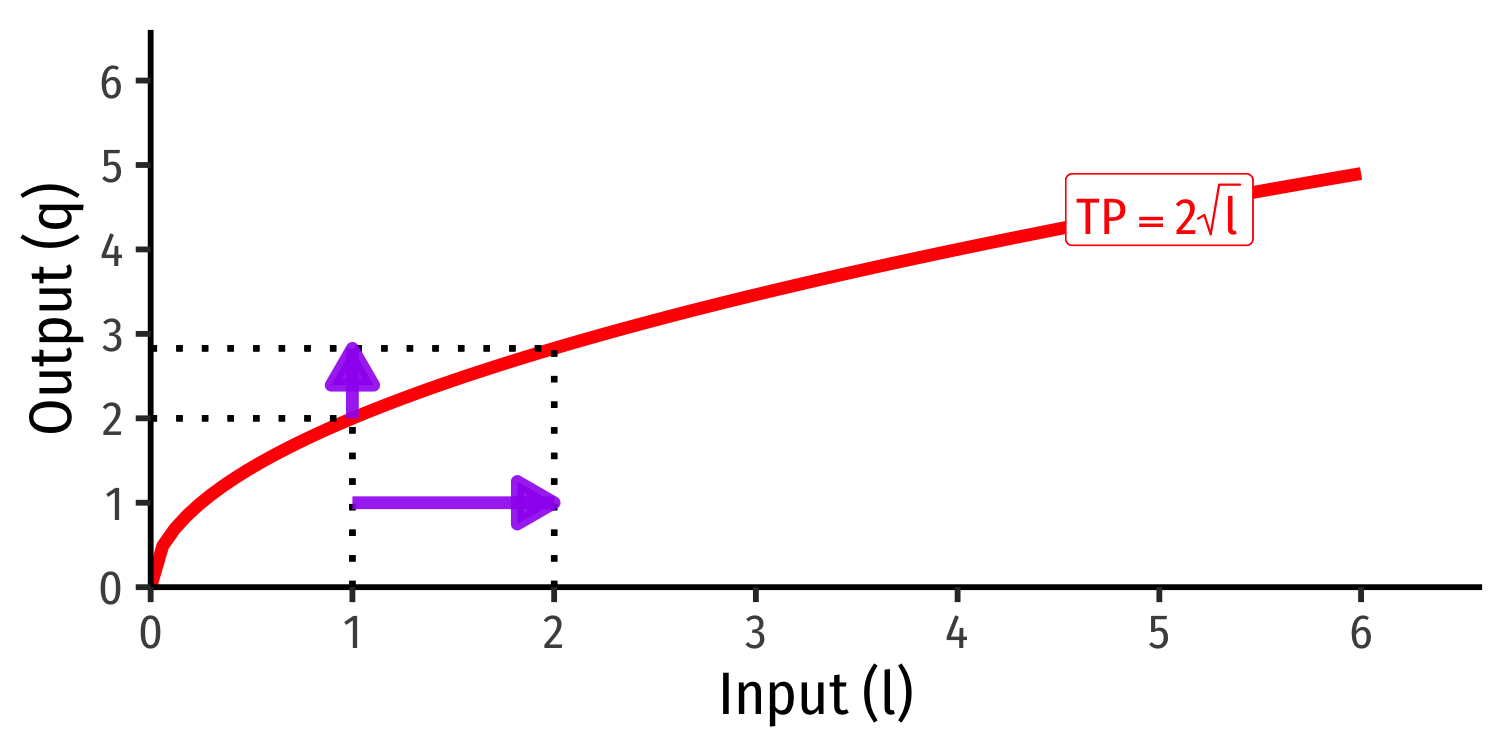

Diminishing Returns
Law of Diminishing Returns: adding more of one factor of production holding all others constant will result in successively lower increases in output
In order to increase output, firm will need to increase all factors!

Average Product of Labor (and Capital)
Average product of labor (APl): total output per worker APl=ql
A measure of labor productivity
Average product of capital (APk): total output per unit of capital APk=qk


The Firm's Problem: Long Run
The Long Run
- In the long run, all factors of production are variable
q=f(k,l)
Can build more factories, open more storefronts, rent more space, invest in machines, etc.
So the firm can choose both l and k
Production Costs are Opportunity Costs
- Remember, economic costs are broader than the common conception of “cost”
- Accounting cost: monetary cost
- Economic cost: value of next best alternative use of resources given up (i.e. opportunity cost)


Production Costs are Opportunity Costs
This leads to the difference between:
- Accounting profit: revenues minus accounting costs
- Economic profit: revenues minus accounting + opportunity costs
A really difficult concept to think about!


Production Costs are Opportunity Costs
- Another helpful perspective:
- Accounting cost: what you historically paid for a resource
- Economic cost: what you can currently get in the market for a selling a resource (it’s value in alternative uses)


A Reminder: It's Demand all the Way Down!
Supply is actually Demand in disguise!
An (opportunity) cost to buy (scarce) inputs for production because other people demand those same inputs to consume or produce other valuable things!
- Price necessary to pull them out of other valuable productive uses in the economy!

Production Costs are Opportunity Costs
Because resources are scarce, and have rivalrous uses, how do we know we are using resources efficiently??
In functioning markets, the market price measures the opportunity cost of using a resource for an alternative use
Firms not only pay for direct use of a resource, but also indirectly compensate society for “pulling the resource out” of alternate uses in the economy!


Production Costs are Opportunity Costs
- Every choice incurs an opportunity cost
Examples:
- If you start a business, you may give up your salary at your current job
- If you invest in a factory, you give up other investment opportunities
- If you use an office building you own, you cannot rent it to other people
- If you hire a skilled worker, you must pay them a high enough salary to deter them from working for other firms

Opportunity Costs vs. Sunk Costs
Opportunity cost is a forward-looking concept
Choices made in the past with non-recoverable costs are called sunk costs
Sunk costs should not enter into future decisions
Many people have difficulty letting go of unchangeable past decisions: sunk cost fallacy

Common Sunk Costs in Business
Licensing fees, long-term lease contracts
Specific capital (with no alternative use): uniforms, menus, signs
Research & Development spending
Advertising spending

The Accounting vs. Economic Point of View I
- Helpful to consider two points of view:
“Accounting point of view”: are you taking in more cash than you are spending?
“Economic point of view”: is your product you making the best social use of your resources
- i.e. are there higher-valued uses of your resources you are keeping them out of?


The Accounting vs. Economic Point of View II
Implications for society: are consumers best off with you using scarce resources (with alternative uses!) to produce your current product?
Remember: this is an economics course, not a business course!
- Economists are pro-market, not pro-business!
- What might be good/bad for one business might have bad/good consequences for society!


Costs in the Short Run
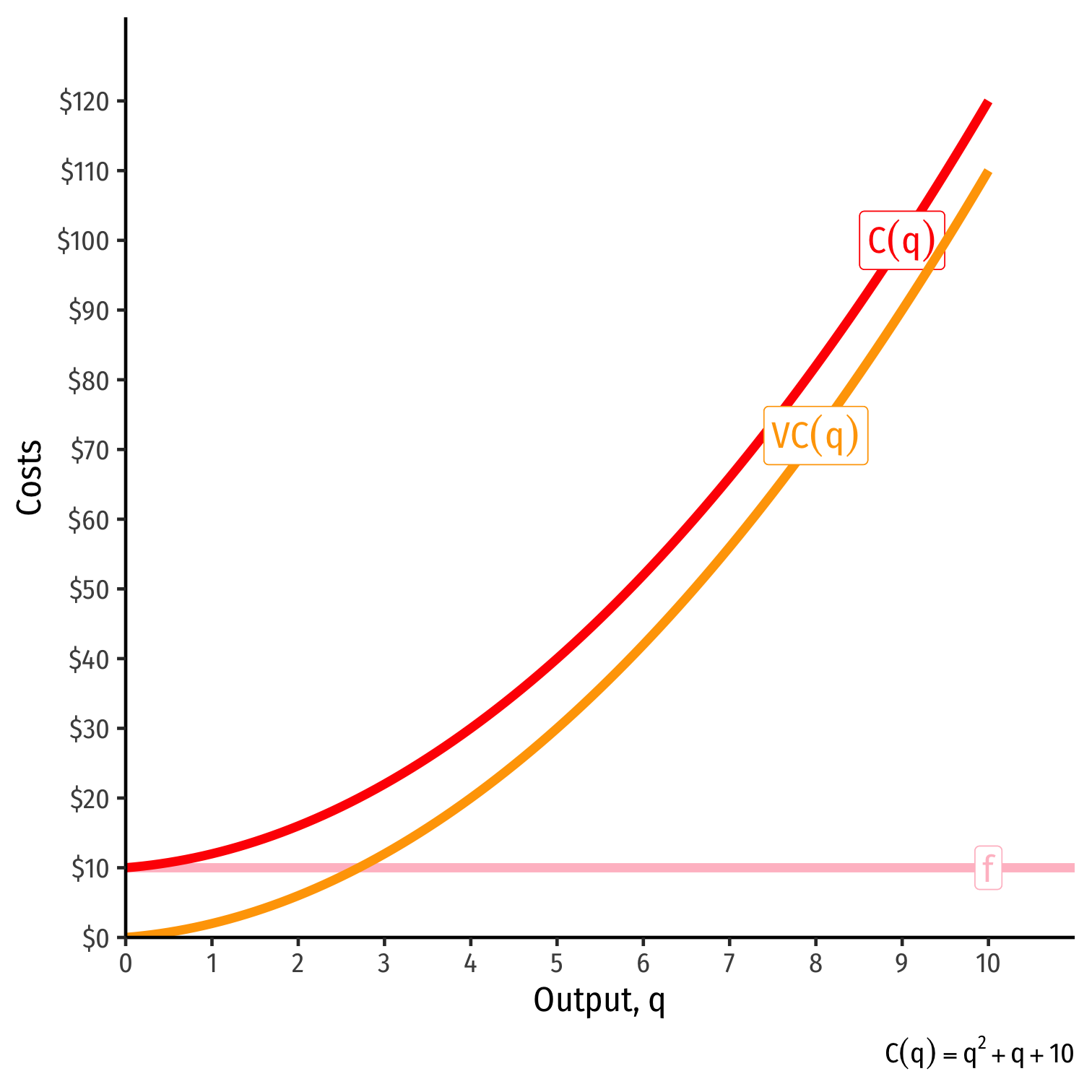
Costs in the Short Run
- Total cost function, C(q) relates output q to the total cost of production C†
C(q)=f+VC(q)
Costs in the Short Run
- Total cost function, C(q) relates output q to the total cost of production C†
C(q)=f+VC(q)
- Two kinds of short run costs:
1. Fixed costs, f are costs that do not vary with output
- Only true in the short run! (Consider this the cost of maintaining your capital)
Costs in the Short Run
- Total cost function, C(q) relates output q to the total cost of production C†
C(q)=f+VC(q)
- Two kinds of short run costs:
1. Fixed costs, f are costs that do not vary with output
- Only true in the short run! (Consider this the cost of maintaining your capital)
2. Variable costs, VC(q) are costs that vary with output (notice the variable in them!)
- Typically, the more production of q, the higher the cost
- e.g. firm is hiring additional labor
† Assuming that (i) firms are always choosing input combinations that minimize total cost and (ii) input prices are constant. See more in today’s appendix.
Fixed vs. Variable costs: Examples

Example: Airlines
Fixed costs: the aircraft, regulatory approval
Variable costs: providing one more flight
Fixed vs. Variable costs: Examples

Example: Car Factory
Fixed costs: the factory, machines in the factory
Variable costs: producing one more car
Fixed vs. Variable costs: Examples

Example: Starbucks
Fixed costs: the retail space, espresso machines
Variable costs: selling one more cup of coffee
Fixed vs. Sunk costs
Diff. between fixed vs. sunk costs?
Sunk costs are a type of fixed cost that are not avoidable or recoverable
Many fixed costs can be avoided or changed in the long run
Common fixed, but not sunk, costs:
- rent for office space, durable equipment, operating permits (that are renewed)
When deciding to stay in business, fixed costs matter, sunk costs do not!

Cost Functions: Example, Visualized
| q | f | VC(q) | C(q) |
|---|---|---|---|
| 0 | 10 | 0 | 10 |
| 1 | 10 | 2 | 12 |
| 2 | 10 | 6 | 16 |
| 3 | 10 | 12 | 22 |
| 4 | 10 | 20 | 30 |
| 5 | 10 | 30 | 40 |
| 6 | 10 | 42 | 52 |
| 7 | 10 | 56 | 66 |
| 8 | 10 | 72 | 82 |
| 9 | 10 | 90 | 100 |
| 10 | 10 | 110 | 120 |
Average Costs
- Average Fixed Cost: fixed cost per unit of output:
AFC(q)=fq
Average Costs
- Average Fixed Cost: fixed cost per unit of output:
AFC(q)=fq
- Average Variable Cost: variable cost per unit of output:
AVC(q)=VC(q)q
Average Costs
- Average Fixed Cost: fixed cost per unit of output:
AFC(q)=fq
- Average Variable Cost: variable cost per unit of output:
AVC(q)=VC(q)q
- Average (Total) Cost: (total) cost per unit of output:
AC(q)=C(q)q
Marginal Cost
- Marginal Cost is the change in total cost for each additional unit of output produced:
MC(q)=ΔC(q)Δq
Calculus: first derivative of the cost function
Marginal cost is the primary cost that matters in making decisions
- All other costs are driven by marginal costs
- This is the main cost that firms can “see”
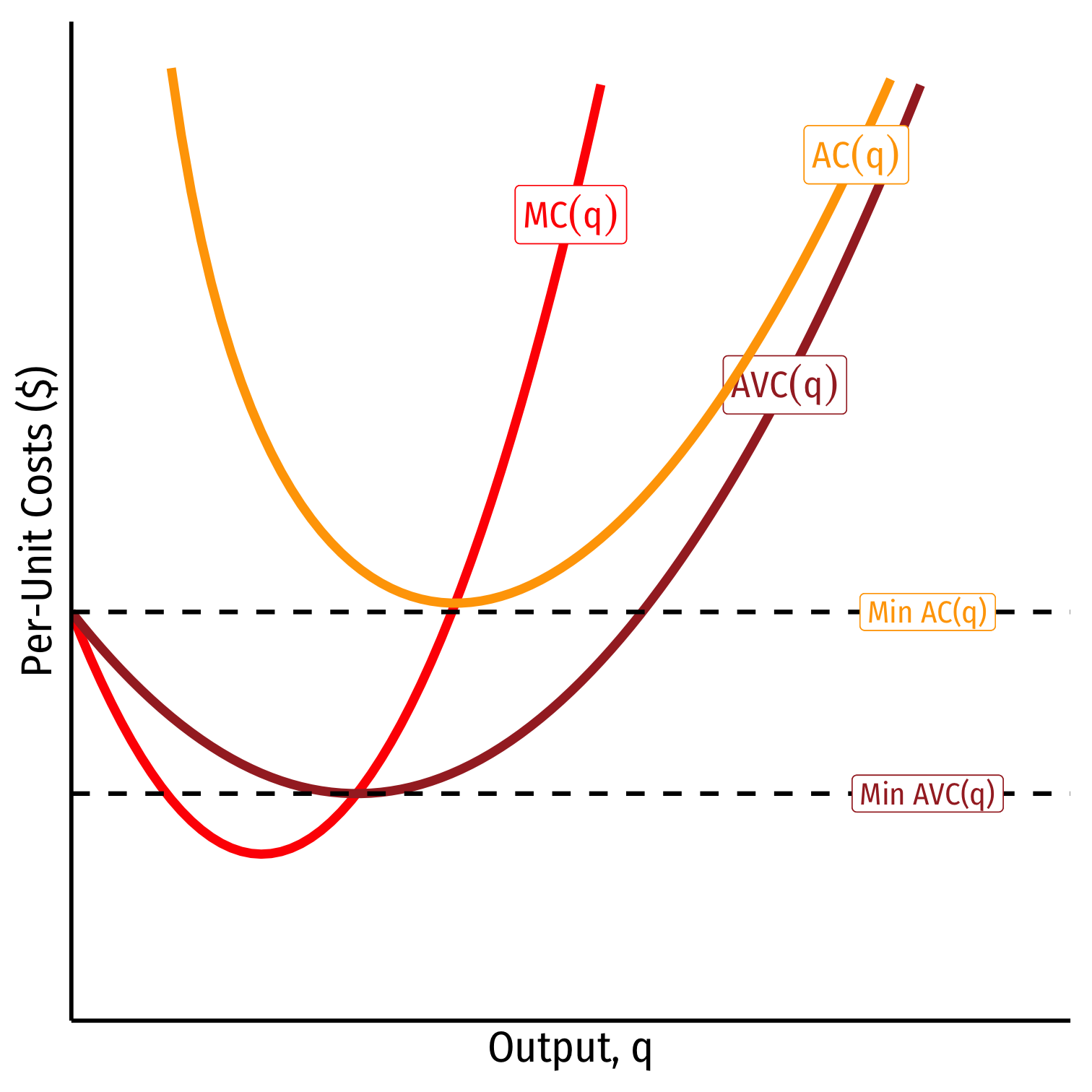
Average and Marginal Costs: Visualized
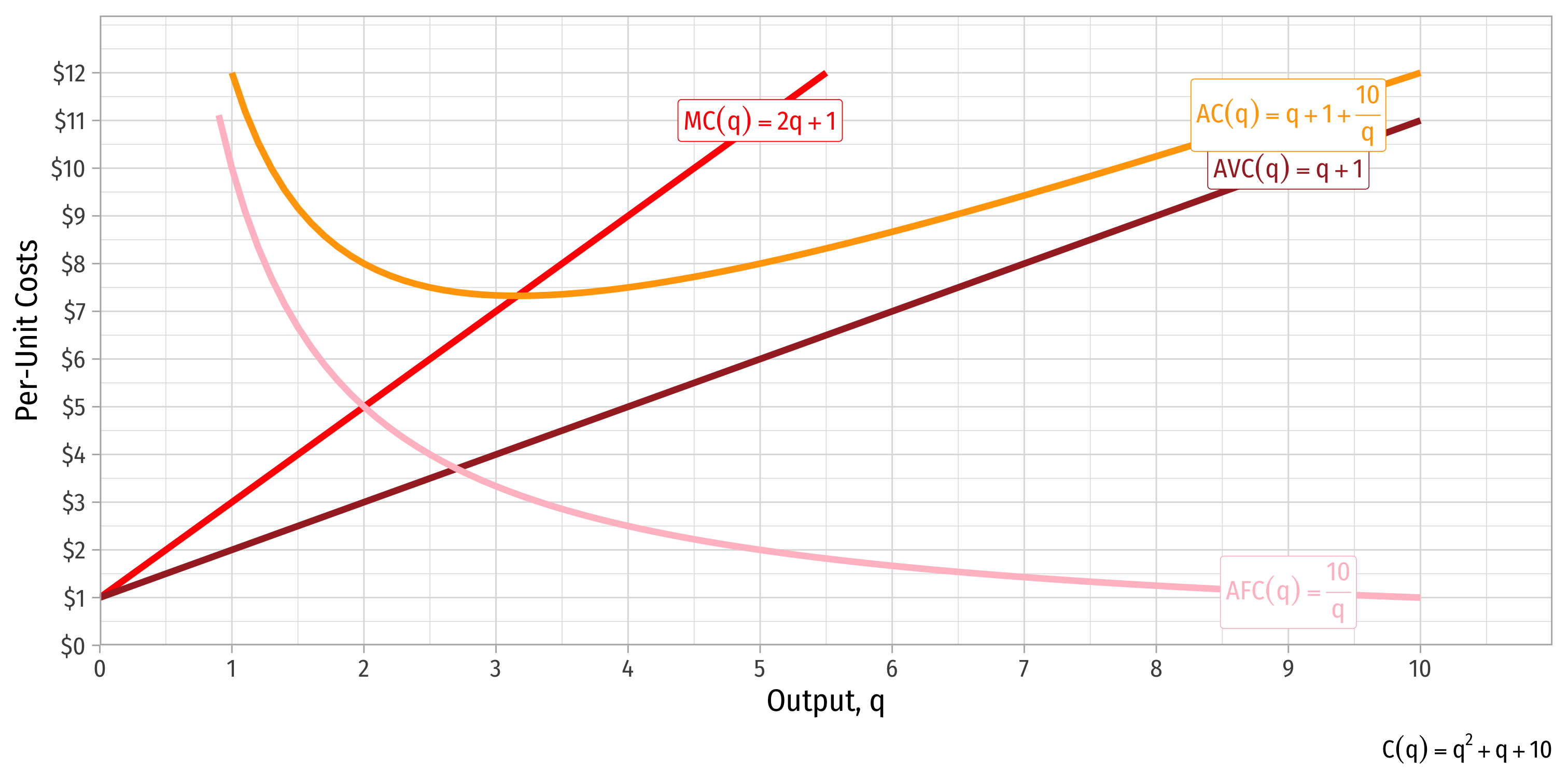
Relationship Between Marginal and Average
Mathematical relationship between a marginal & an average value
If marginal < average, then average ↓
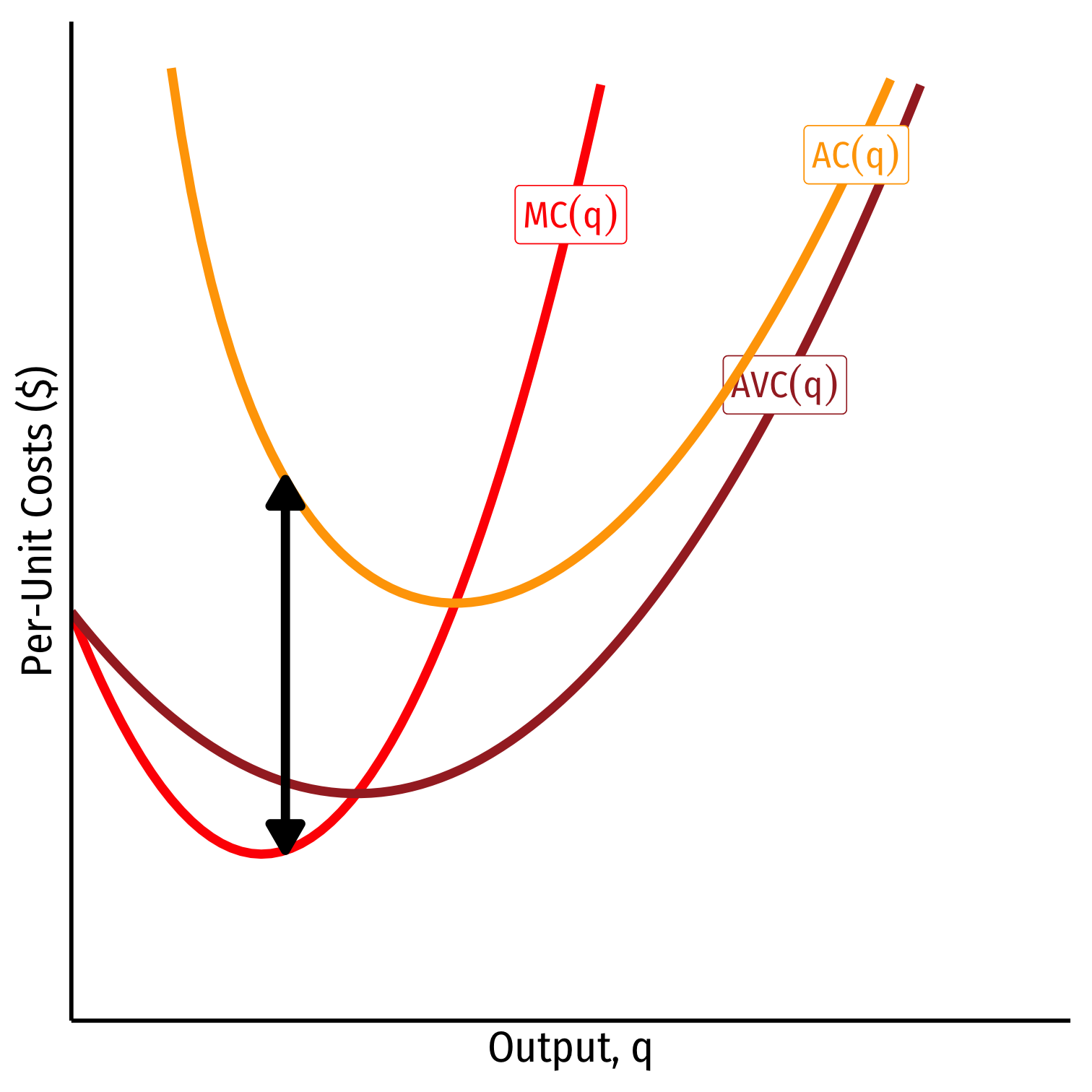
Relationship Between Marginal and Average
Mathematical relationship between a marginal & an average value
If marginal < average, then average ↓
If marginal > average, then average ↑
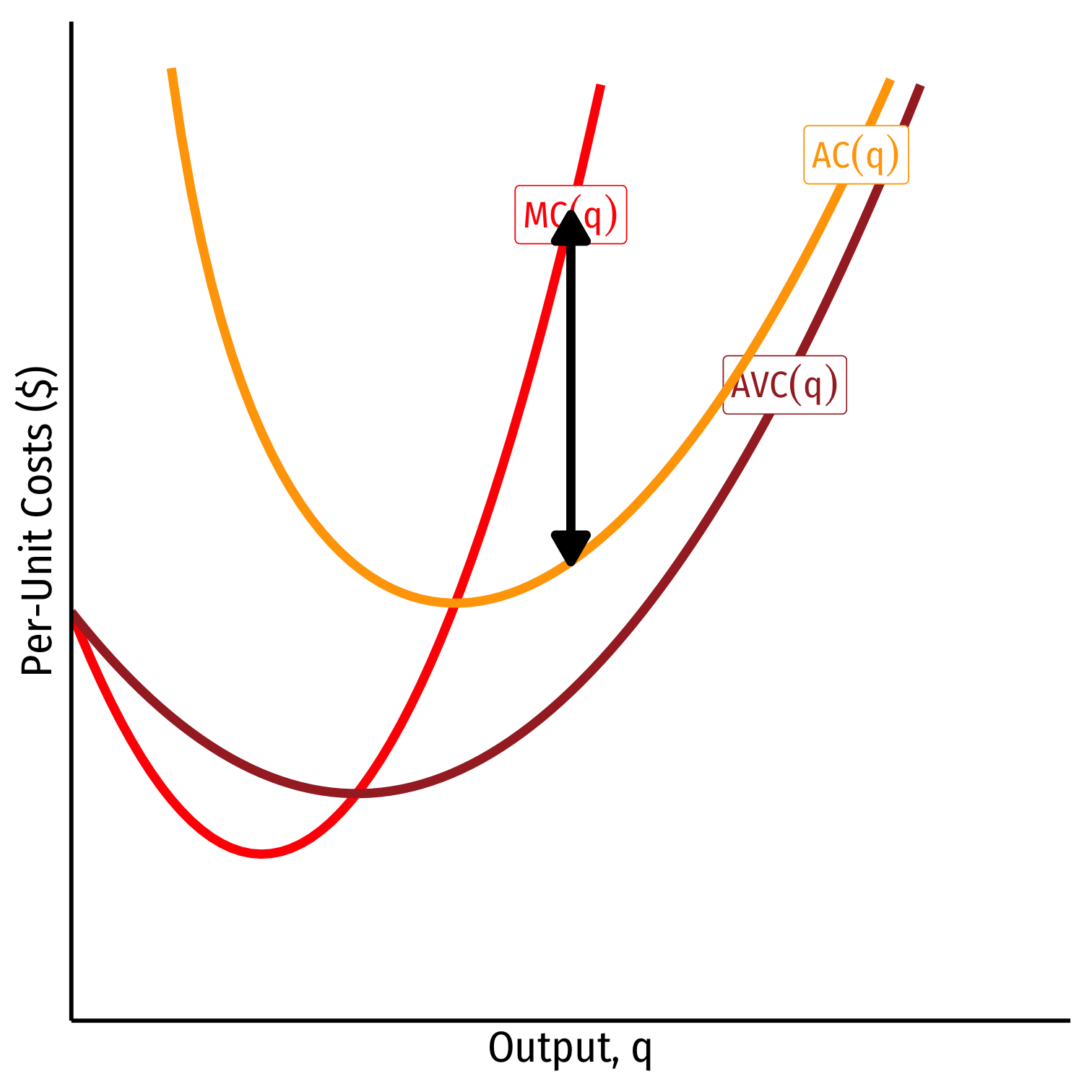
Relationship Between Marginal and Average
Mathematical relationship between a marginal & an average value
If marginal < average, then average ↓
If marginal > average, then average ↑
When marginal = average, average is maximized/minimized
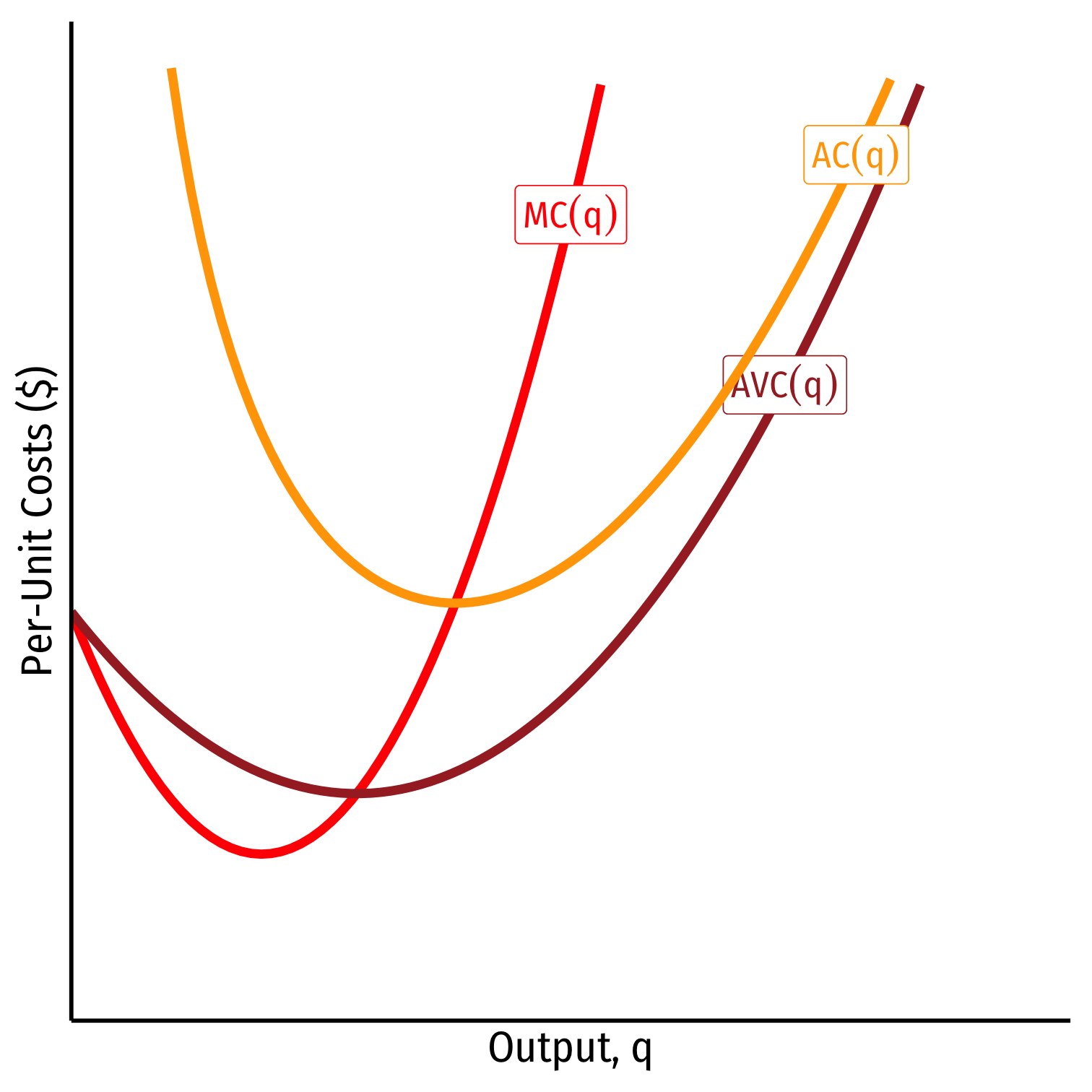
Relationship Between Marginal and Average
Mathematical relationship between a marginal & an average value
If marginal < average, then average ↓
If marginal > average, then average ↑
When marginal = average, average is maximized/minimized
- When MC(q)=AC(q), AC(q) is at a minimum (break-even price)
- When MC(q)=AVC(q), AVC(q) is at a minimum (shut-down price)
Costs in the Long Run
Costs in the Long Run
Long run: firm can change all factors of production & vary scale of production
Long run average cost, LRAC(q): cost per unit of output when the firm can change both l and k to make more q
Long run marginal cost, LRMC(q): change in long run total cost as the firm produce an additional unit of q (by changing both l and/or k)

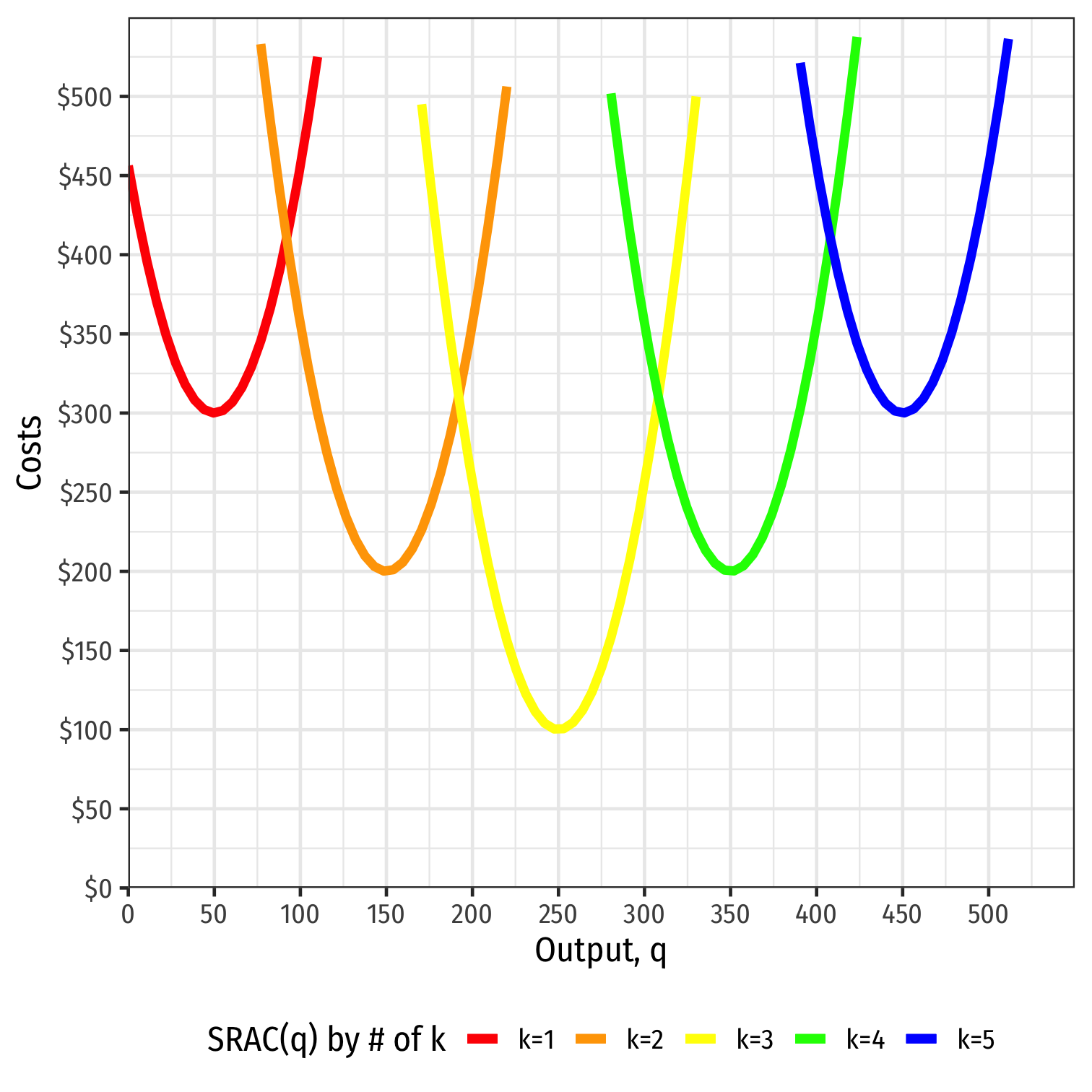
Average Cost in the Long Run
Long run: firm can choose k (factories, locations, etc)
Separate short run average cost (SRAC) curves for each amount of k potentially chosen
Average Cost in the Long Run
Long run: firm can choose k (factories, locations, etc)
Separate short run average cost (SRAC) curves for each amount of k potentially chosen
Long run average cost (LRAC) curve “envelopes” the lowest (optimal) regions of all the SRAC curves!
“Subject to producing the optimal amount of output, choose l and k to minimize cost”
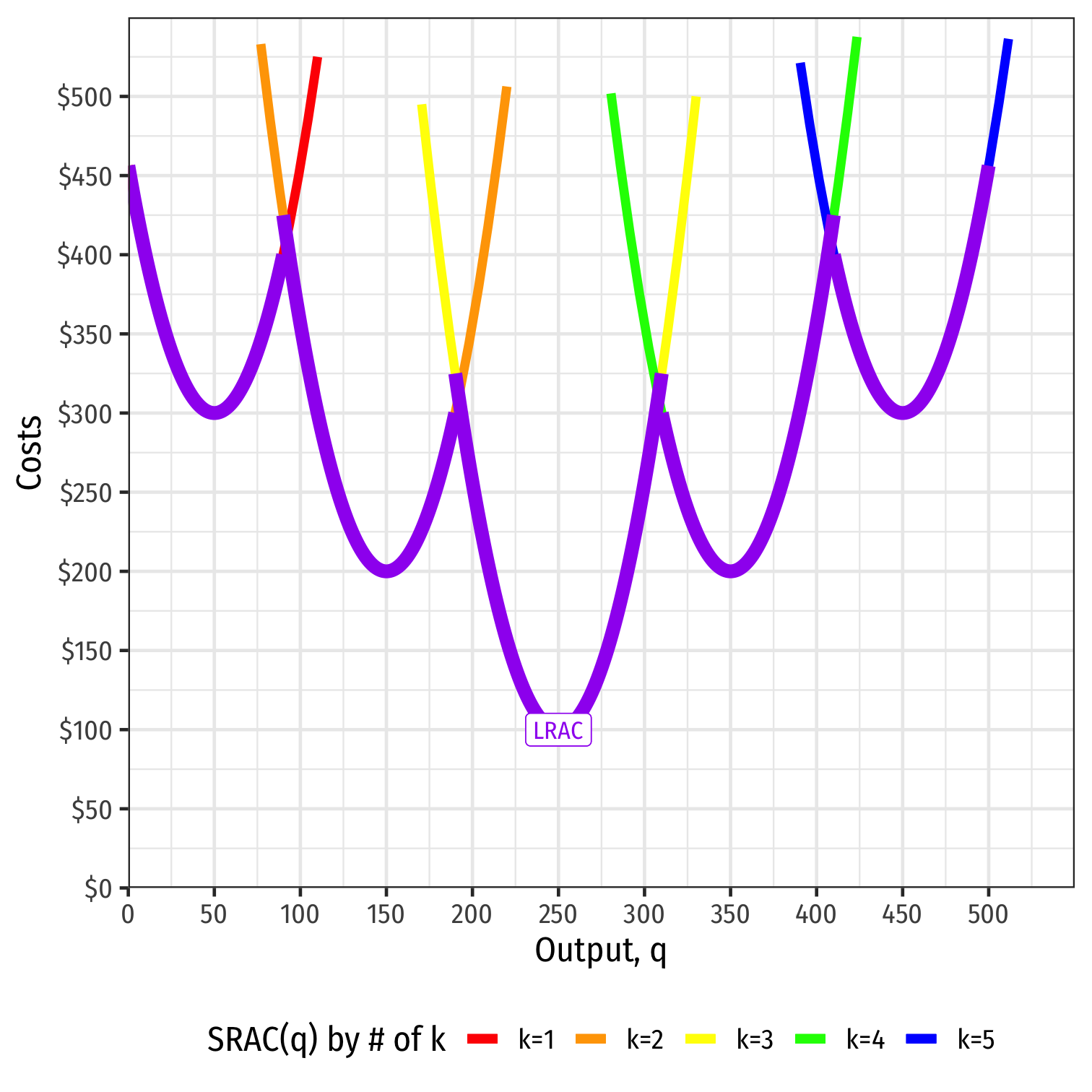
Long Run Costs & Scale Economies I
Further important properties about costs based on scale economies of production: change in average costs when output is increased (scaled)
Economies of scale: average costs fall with more output
- High fixed costs AFC>AVC(q) low variable costs
Diseconomies of scale: average costs rise with more output
- Low fixed costs AFC<AVC(q) high variable costs
Constant economies of scale: average costs don’t change with more output
- Firm at minimum average cost (optimal plant size), called minimum efficient scale (MES)
Long Run Costs & Scale Economies II
- Minimum Efficient Scale: q with the lowest AC(q)
- “optimal firm size”

Long Run Costs & Scale Economies II
Minimum Efficient Scale: q with the lowest AC(q)
- “optimal firm size”
Economies of Scale: ↑q, ↓AC(q)
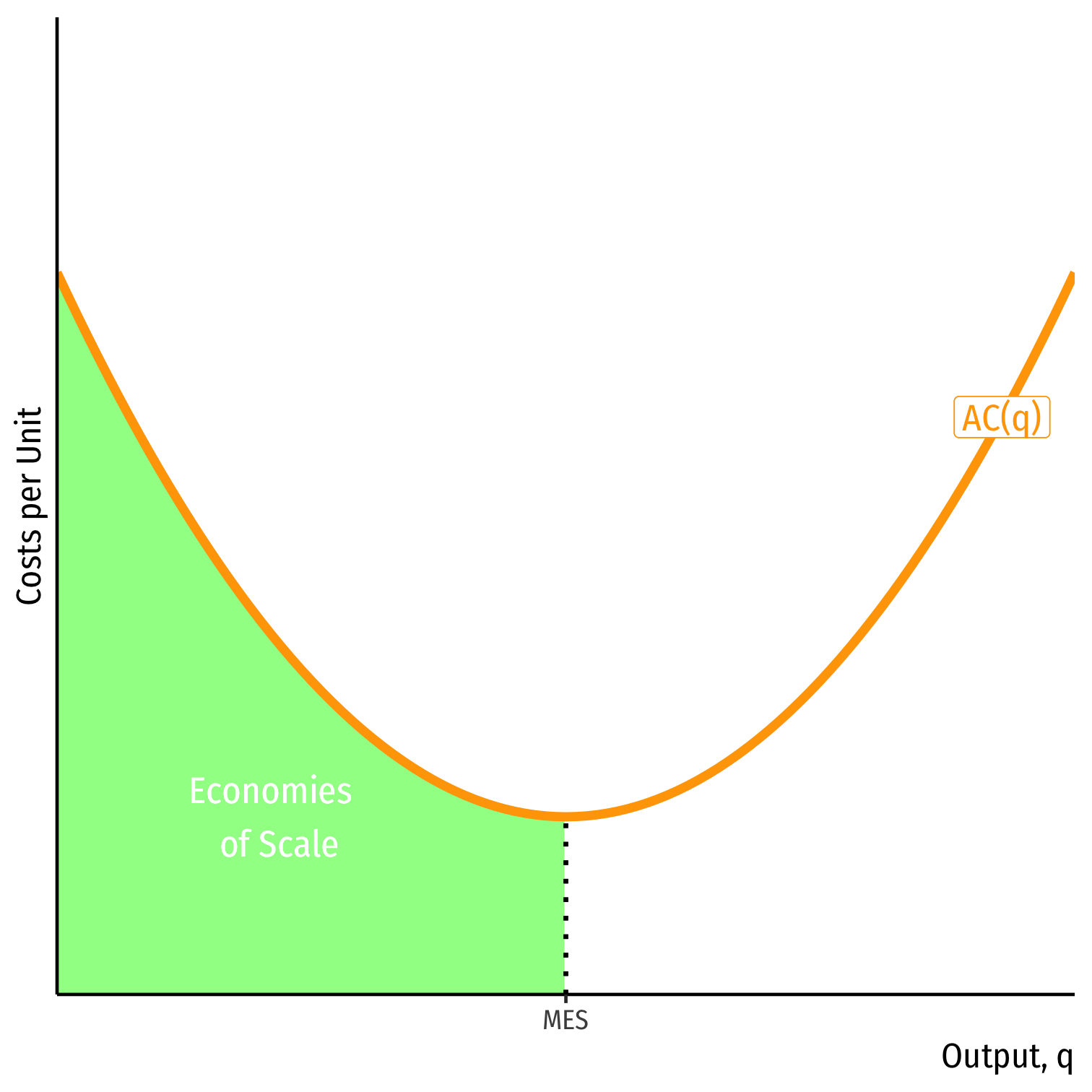
Long Run Costs & Scale Economies II
Minimum Efficient Scale: q with the lowest AC(q)
- “optimal firm size”
Economies of Scale: ↑q, ↓AC(q)
Diseconomies of Scale: ↑q, ↑AC(q)
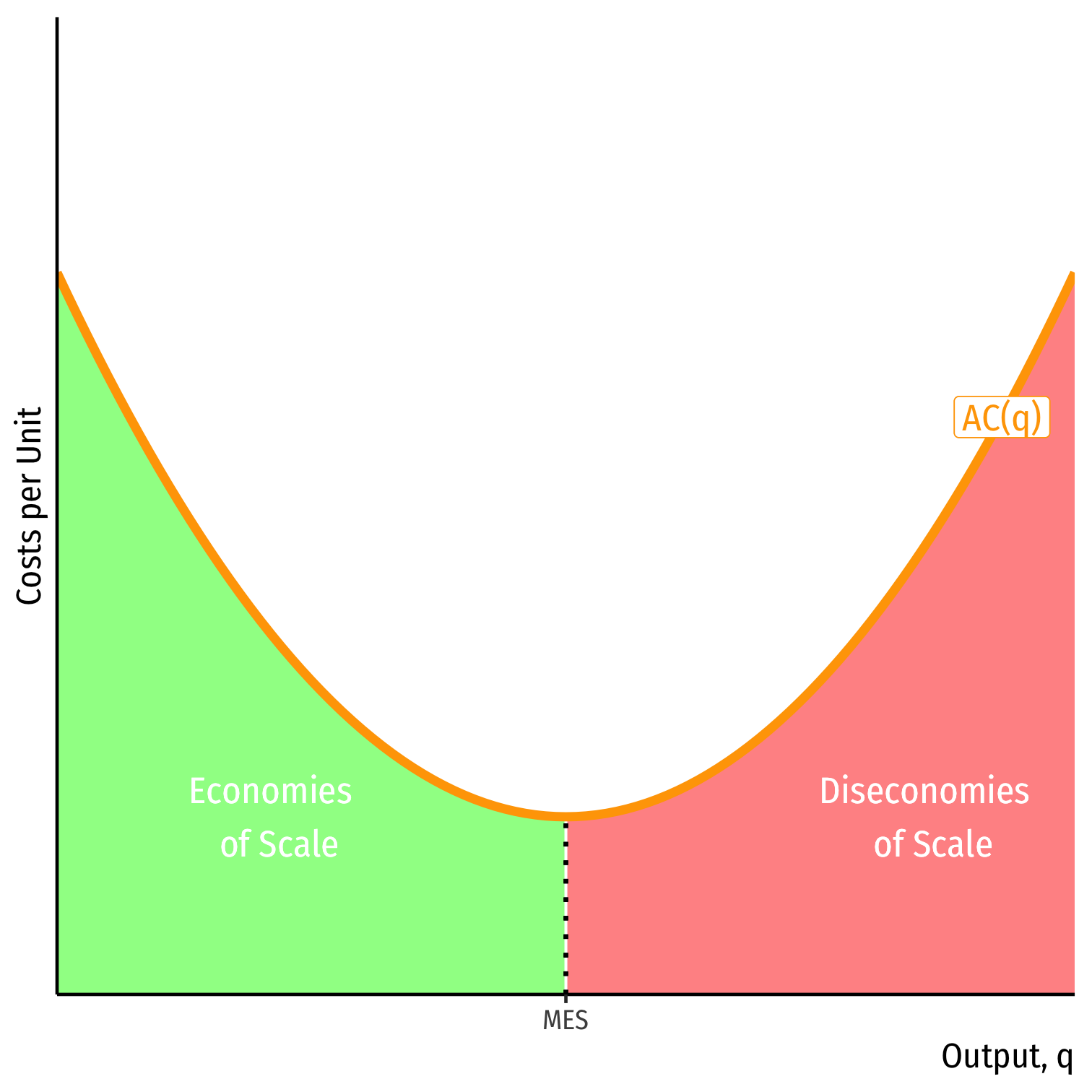
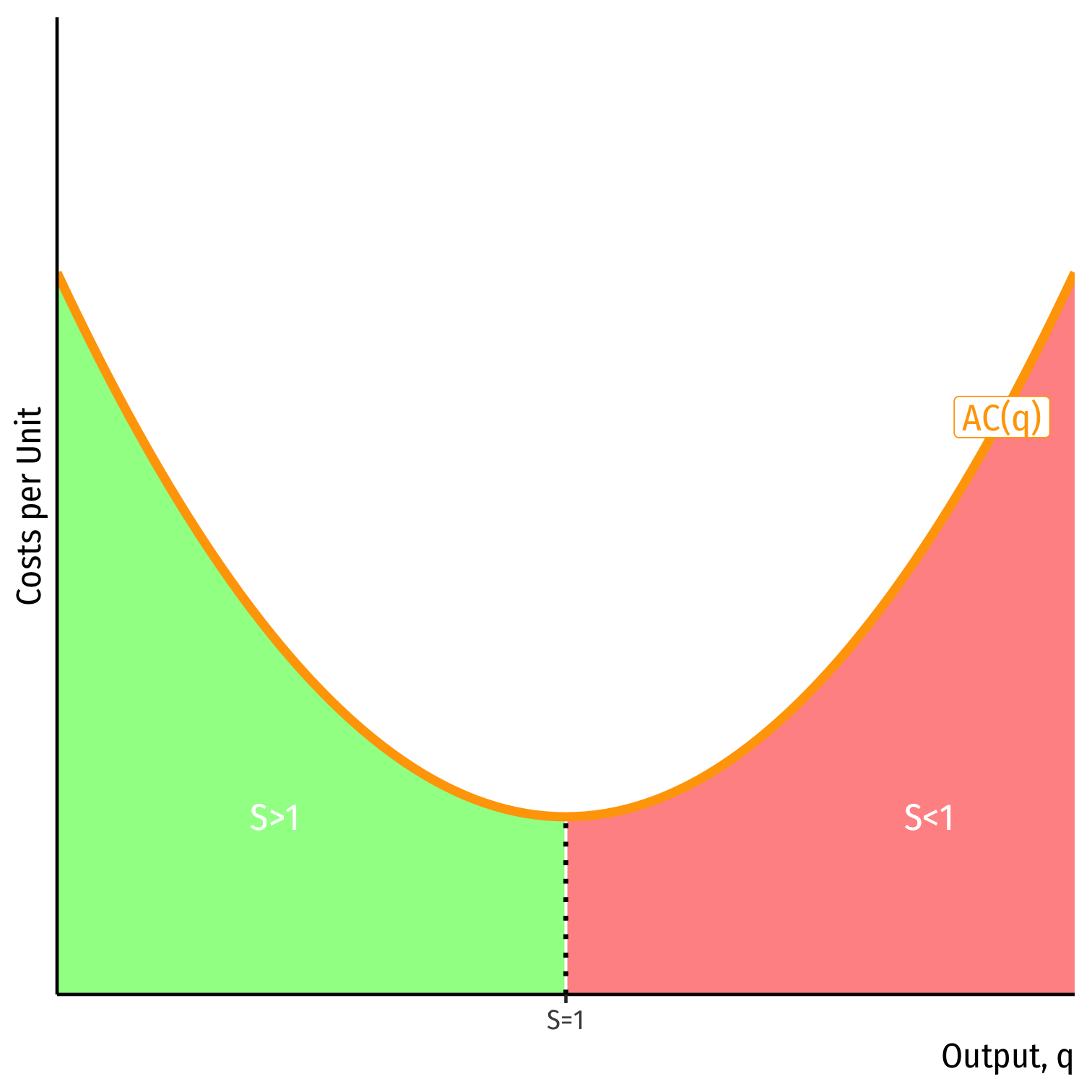
Long Run Costs & Scale Economies III
- Can measure economies of scale (S):
S(q)=AC(q)MC(q)
- S>1: economies of scale at q
- S<1: diseconomies of scale at q
- S=1: minimum efficient scale at q
Economies of Scope
We often assume single-product plants/firms, but in reality most firms/plants are multi-product
Economies of Scope: cost of producing multiple products (e.g. q1 and q2) in a single plant exceeds costs of producing a single product in each plant
C(q1,q2)<C(q1,0)+C(0,q2)

Revenues
Revenues for Firms in Competitive Industries I
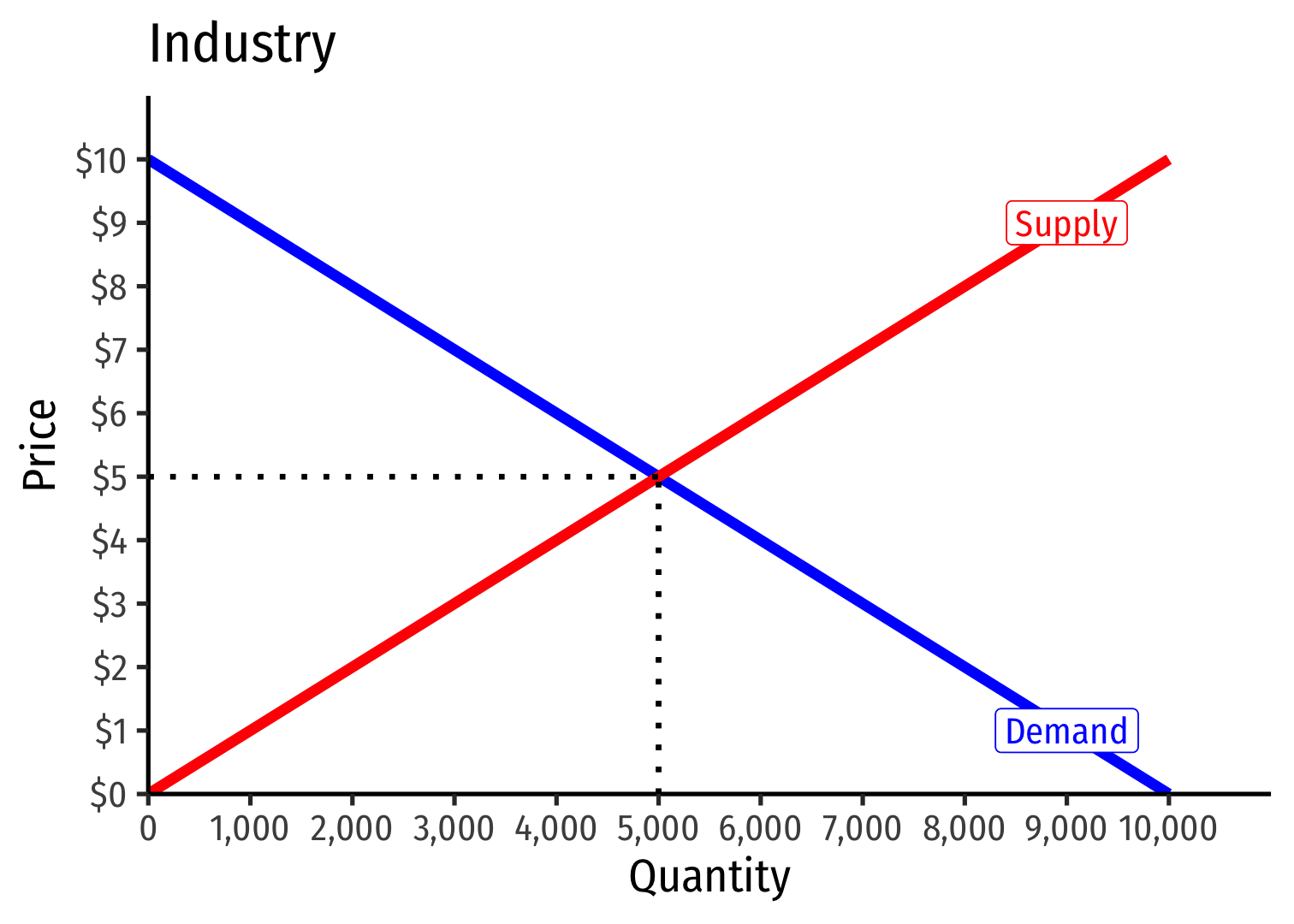
Revenues for Firms in Competitive Industries I


Revenues for Firms in Competitive Industries I


- Demand for a firm’s product is perfectly elastic at the market price
Revenues for Firms in Competitive Industries I


Demand for a firm’s product is perfectly elastic at the market price
Where did the supply curve come from? You’ll know today
Revenues for Firms in Competitive Industries II

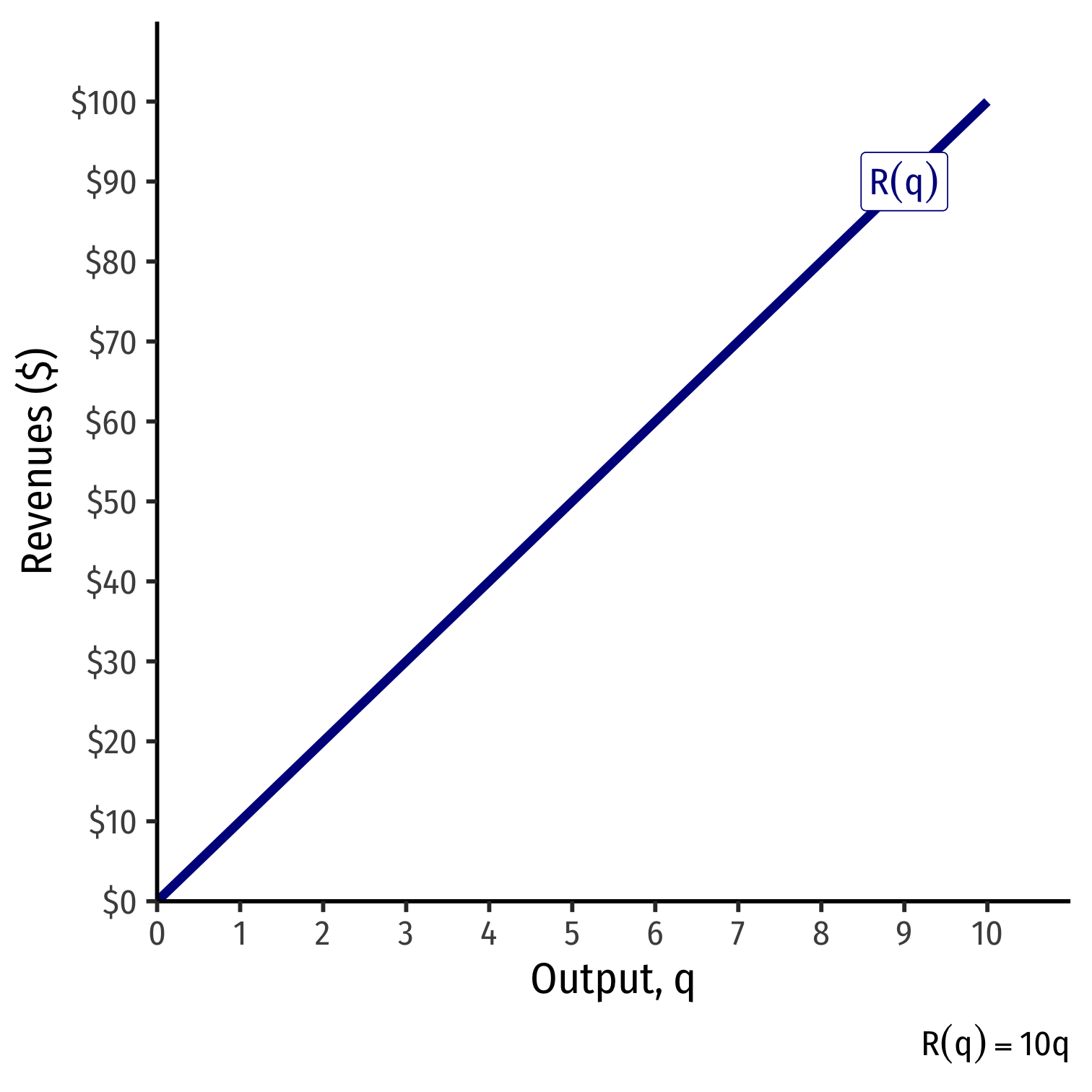
- Total Revenue R(q)=pq
Average and Marginal Revenues
- Average Revenue: revenue per unit of output
AR(q)=Rq
- AR(q) is by definition equal to the price! (Why?)
Average and Marginal Revenues
Average Revenue: revenue per unit of output AR(q)=Rq
- AR(q) is by definition equal to the price! (Why?)
Marginal Revenue: change in revenues for each additional unit of output sold: MR(q)=ΔR(q)Δq
- Calculus: first derivative of the revenues function
- For a competitive firm (only), MR(q) = p, i.e. the price!
Average and Marginal Revenues: Example
Example: A firm sells bushels of wheat in a very competitive market. The current market price is $10/bushel.
Average and Marginal Revenues: Example
Example: A firm sells bushels of wheat in a very competitive market. The current market price is $10/bushel.
For the 1st bushel sold:
What is the total revenue?
What is the average revenue?
Average and Marginal Revenues: Example
Example: A firm sells bushels of wheat in a very competitive market. The current market price is $10/bushel.
For the 1st bushel sold:
What is the total revenue?
What is the average revenue?
For the 2nd bushel sold:
What is the total revenue?
What is the average revenue?
What is the marginal revenue?
Total Revenue, Example: Visualized
| q | R(q) |
|---|---|
| 0 | 0 |
| 1 | 10 |
| 2 | 20 |
| 3 | 30 |
| 4 | 40 |
| 5 | 50 |
| 6 | 60 |
| 7 | 70 |
| 8 | 80 |
| 9 | 90 |
| 10 | 100 |
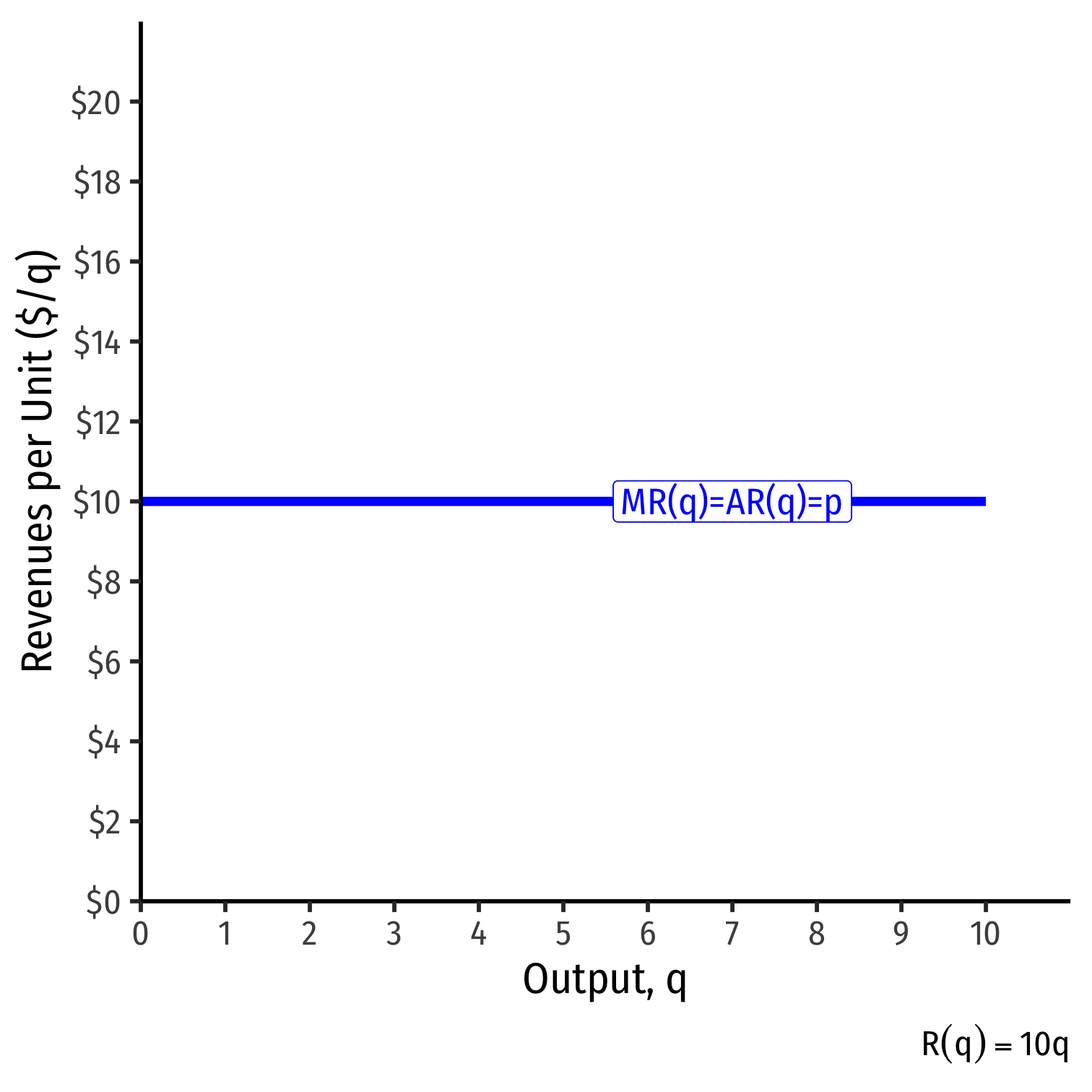
Average and Marginal Revenue, Example: Visualized
| q | R(q) | AR(q) | MR(q) |
|---|---|---|---|
| 0 | 0 | − | − |
| 1 | 10 | 10 | 10 |
| 2 | 20 | 10 | 10 |
| 3 | 30 | 10 | 10 |
| 4 | 40 | 10 | 10 |
| 5 | 50 | 10 | 10 |
| 6 | 60 | 10 | 10 |
| 7 | 70 | 10 | 10 |
| 8 | 80 | 10 | 10 |
| 9 | 90 | 10 | 10 |
| 10 | 100 | 10 | 10 |